Homogeneous partial differential equation examples pdf Morrisburg

PARTIAL DIFFERENTIAL EQUATIONS mat.iitm.ac.in We study a homogeneous partial differential equation and get its entire solutions represented in convergent series of Laguerre polynomials. Moreover, the formulae of the order and type of the solutions are established. In this paper, we concentrate on the following partial differential equation (PDE

20.pdf Partial Differential Equation Ordinary

Lecture Notes PDE 1 Partial Differential Equation. Partial Differential Equations This chapter introduces basic concepts and definitions for partial differential equations (PDEs) and solutions to a variety of PDEs., example the pendulum equation, (1.1) d2Θ dt2 + g L sinΘ = 0, describes the angle, Θ, a pendulum makes with the vertical as a function of time, t. Here g and L are constants (the acceleration due to gravity and length of the pendulum respectively), tis the independent variable and Θ is the dependent variable. This is an ODE because there is only one independent variable, here t which.

An example of a PDE: tension in the string In this case: = = = ПЃ ПЃ T T c2. Background to this course Partial Differential Equations Partial differentiation Ordinary Differential Equations Fourier series Numerical methods Vector calculus Electrical engineering Mechanical engineering Civil engineering Biomedical We now give brief reminders of partial differentiation, engineering ODEs, and Homogeneous PDE: If all the terms of a PDE contains the dependent variable or its partial derivatives then such a PDE is called non-homogeneous partial differential equation or homogeneous otherwise. In the above six examples eqn 6.1.6 is non-homogeneous where as the first five equations are homogeneous.

The homogeneous Laplace equation, uxx + uyy = 0, can be thought of as a special case of the wave and heat equation where the function u(x,y,t) is independent of t. We study a homogeneous partial differential equation and get its entire solutions represented in convergent series of Laguerre polynomials. Moreover, the formulae of the order and type of the solutions are established. In this paper, we concentrate on the following partial differential equation (PDE

6/11/2015 · This video lecture " Homogeneous Linear Partial Differential Equation With Constant Coefficient- Complementary Function in Hindi" will help students to understand following topic of … 1 Introduction 1.1 Preliminaries A partial differential equation (PDE) describes a relation between an unknown function and its partial derivatives.

Second Order Differential Equations is constant coefficient and homogeneous. In this example the dependent variable is x. (d) is constant coefficient and homogeneous. Note: Acomplementaryfunction is the generalsolution of ahomogeneous, lineardifferential equation. HELM (2008): Section 19.3: Second Order Differential Equations 31. 2. Finding the complementary function … 7/11/2015 · This video lecture " Homogeneous Linear Partial Differential Equation With Constant Coefficient- CF and PI in Hindi" will help students to understand following topic of unit-IV …

The homogeneous Laplace equation, uxx + uyy = 0, can be thought of as a special case of the wave and heat equation where the function u(x,y,t) is independent of t. 1 To determine the general solution to homogeneous second order differential equation: y " p (x )y ' q (x)y 0 Find two linearly independent solutions

20/06/2011 · In this video, I solve a homogeneous differential equation by using a change of variables. This example is looooong, requiring partial fractions to integrate! So much fun though! 6/11/2015 · This video lecture " Homogeneous Linear Partial Differential Equation With Constant Coefficient- Complementary Function in Hindi" will help students to understand following topic of …

In order to solve this equation, let's consider that the solution to the homogeneous equation will allow us to obtain a system of basis functions that satisfy the given boundary conditions. We start with the Laplace equation: u x x + u y y = 0 . {\displaystyle u_{xx}+u_{yy}=0~.} GEOMETRY OF A SYSTEM OF LINEAR HOMOGENEOUS PARTIAL DIFFERENTIAL EQUATIONS By Raymundo A. Fauila Introduction Using a system of partial differential equations, in this paper we shall study a configuration of surfaces and congruences of lines

A linear partial di erential equation is non-homogeneous if it contains a term that does not depend on the dependent variable. For example, consider the wave equation The homogeneous Laplace equation, uxx + uyy = 0, can be thought of as a special case of the wave and heat equation where the function u(x,y,t) is independent of t.

20/06/2011В В· In this video, I solve a homogeneous differential equation by using a change of variables. This example is looooong, requiring partial fractions to integrate! So much fun though! A linear partial di erential equation is non-homogeneous if it contains a term that does not depend on the dependent variable. For example, consider the wave equation

We study a homogeneous partial differential equation and get its entire solutions represented in convergent series of Laguerre polynomials. Moreover, the formulae of the order and type of the solutions are established. In this paper, we concentrate on the following partial differential equation (PDE Homogeneous PDE: If all the terms of a PDE contains the dependent variable or its partial derivatives then such a PDE is called non-homogeneous partial differential equation or homogeneous otherwise. In the above six examples eqn 6.1.6 is non-homogeneous where as the first five equations are homogeneous.

Chapter 12 Partial Differential Equations. An example of a PDE: tension in the string In this case: = = = ρ ρ T T c2. Background to this course Partial Differential Equations Partial differentiation Ordinary Differential Equations Fourier series Numerical methods Vector calculus Electrical engineering Mechanical engineering Civil engineering Biomedical We now give brief reminders of partial differentiation, engineering ODEs, and, Examples 1. Partial differential equations A partial differential equation (PDE) is an equation giving a relation between a function of two or more variables, u,and its partial derivatives. The order of the PDE is the order of the highest partial derivative of u that appears in the PDE. APDEislinear if it is linear in u and in its partial derivatives. A linear PDE is homogeneous if all of.

Partial Differential Equations engr.usask.ca

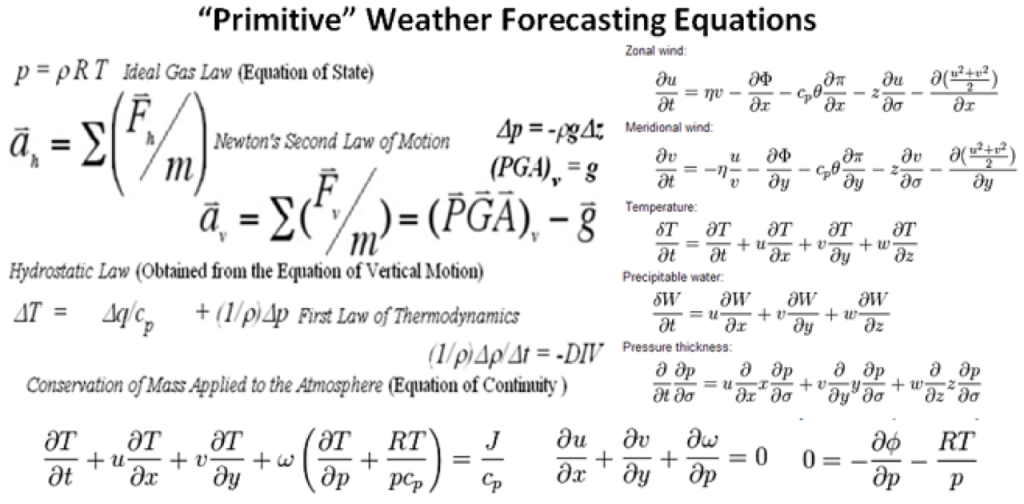
Partial Differential Equations engr.usask.ca. We study a homogeneous partial differential equation and get its entire solutions represented in convergent series of Laguerre polynomials. Moreover, the formulae of the order and type of the solutions are established. In this paper, we concentrate on the following partial differential equation (PDE, 6/11/2015 · This video lecture " Homogeneous Linear Partial Differential Equation With Constant Coefficient- Complementary Function in Hindi" will help students to understand following topic of ….

Chapter 12 Partial Differential Equations. Nonhomogeneous differential equations are the same as homogeneous differential equations, except they can have terms involving only x (and constants) on the right side, as in this equation: You also can write nonhomogeneous differential equations in this format: y ” + p ( x ) y ‘ + q ( x ) y = g ( x )., Nonhomogeneous differential equations are the same as homogeneous differential equations, except they can have terms involving only x (and constants) on the right side, as in this equation: You also can write nonhomogeneous differential equations in this format: y ” + p ( x ) y ‘ + q ( x ) y = g ( x )..

LINEAR HOMOGENEOUS PARTIAL DIFFERENTIAL EQUATIONS

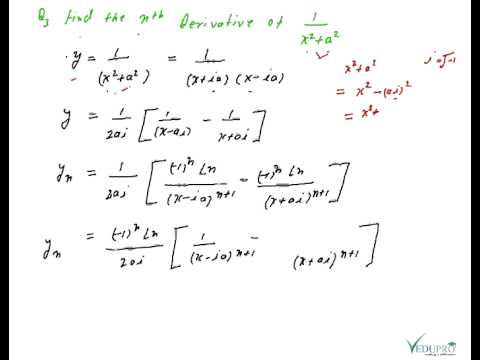
PARTIAL DIFFERENTIAL EQUATIONS mat.iitm.ac.in. Second Order Differential Equations is constant coefficient and homogeneous. In this example the dependent variable is x. (d) is constant coefficient and homogeneous. Note: Acomplementaryfunction is the generalsolution of ahomogeneous, lineardifferential equation. HELM (2008): Section 19.3: Second Order Differential Equations 31. 2. Finding the complementary function … The best solution strategy for differential equations depends on their order and whether they are ordinary or partial, linear or non-linear, and homogeneous or heterogeneous..

Change of Variables / Homogeneous Differential Equation

Classification of differential equations Maths for

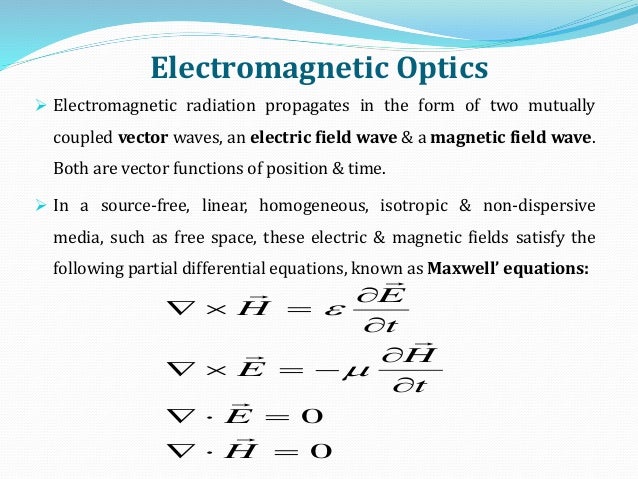
Partial Differential Equations This chapter introduces basic concepts and definitions for partial differential equations (PDEs) and solutions to a variety of PDEs. 6/11/2015 · This video lecture " Homogeneous Linear Partial Differential Equation With Constant Coefficient- Complementary Function in Hindi" will help students to understand following topic of …

An example of a PDE: tension in the string In this case: = = = ρ ρ T T c2. Background to this course Partial Differential Equations Partial differentiation Ordinary Differential Equations Fourier series Numerical methods Vector calculus Electrical engineering Mechanical engineering Civil engineering Biomedical We now give brief reminders of partial differentiation, engineering ODEs, and example the pendulum equation, (1.1) d2Θ dt2 + g L sinΘ = 0, describes the angle, Θ, a pendulum makes with the vertical as a function of time, t. Here g and L are constants (the acceleration due to gravity and length of the pendulum respectively), tis the independent variable and Θ is the dependent variable. This is an ODE because there is only one independent variable, here t which

Example Homogeneous equations Linear polynomial di erential operators In our example, y00+y0 6y = 0; with auxiliary polynomial P(r) = r2 +r 6; the roots of P(r) are r = 2 and r = 3. An equivalent. Higher Order Linear Di erential Equations Math 240 Linear DE Linear di erential operators Familiar stu Example Homogeneous equations :: Higher Order Linear Di erential Equations Math 240 Linear DE The homogeneous Laplace equation, uxx + uyy = 0, can be thought of as a special case of the wave and heat equation where the function u(x,y,t) is independent of t.

A linear partial di erential equation is non-homogeneous if it contains a term that does not depend on the dependent variable. For example, consider the wave equation Homogeneous PDE: If all the terms of a PDE contains the dependent variable or its partial derivatives then such a PDE is called non-homogeneous partial differential equation or homogeneous otherwise. In the above six examples eqn 6.1.6 is non-homogeneous where as the first five equations are homogeneous.

1 To determine the general solution to homogeneous second order differential equation: y " p (x )y ' q (x)y 0 Find two linearly independent solutions The best solution strategy for differential equations depends on their order and whether they are ordinary or partial, linear or non-linear, and homogeneous or heterogeneous.

1 Introduction 1.1 Preliminaries A partial differential equation (PDE) describes a relation between an unknown function and its partial derivatives. A linear partial di erential equation is non-homogeneous if it contains a term that does not depend on the dependent variable. For example, consider the wave equation

example the pendulum equation, (1.1) d2Θ dt2 + g L sinΘ = 0, describes the angle, Θ, a pendulum makes with the vertical as a function of time, t. Here g and L are constants (the acceleration due to gravity and length of the pendulum respectively), tis the independent variable and Θ is the dependent variable. This is an ODE because there is only one independent variable, here t which Second Order Differential Equations is constant coefficient and homogeneous. In this example the dependent variable is x. (d) is constant coefficient and homogeneous. Note: Acomplementaryfunction is the generalsolution of ahomogeneous, lineardifferential equation. HELM (2008): Section 19.3: Second Order Differential Equations 31. 2. Finding the complementary function …

example the pendulum equation, (1.1) d2Θ dt2 + g L sinΘ = 0, describes the angle, Θ, a pendulum makes with the vertical as a function of time, t. Here g and L are constants (the acceleration due to gravity and length of the pendulum respectively), tis the independent variable and Θ is the dependent variable. This is an ODE because there is only one independent variable, here t which 7/11/2015 · This video lecture " Homogeneous Linear Partial Differential Equation With Constant Coefficient- CF and PI in Hindi" will help students to understand following topic of unit-IV …

20/06/2011В В· In this video, I solve a homogeneous differential equation by using a change of variables. This example is looooong, requiring partial fractions to integrate! So much fun though! We study a homogeneous partial differential equation and get its entire solutions represented in convergent series of Laguerre polynomials. Moreover, the formulae of the order and type of the solutions are established. In this paper, we concentrate on the following partial differential equation (PDE

20.pdf - Free download as PDF File (.pdf), Text File (.txt) or read online for free. Scribd is the world's largest social reading and publishing site. Search Search Example 6: The differential equation is homogeneous because both M ( x,y ) = x 2 – y 2 and N ( x,y ) = xy are homogeneous functions of the same degree (namely, 2). The method for solving homogeneous equations follows from this fact:

Second Order Differential Equations is constant coefficient and homogeneous. In this example the dependent variable is x. (d) is constant coefficient and homogeneous. Note: Acomplementaryfunction is the generalsolution of ahomogeneous, lineardifferential equation. HELM (2008): Section 19.3: Second Order Differential Equations 31. 2. Finding the complementary function … Partial Differential Equations Following are some classical partial differential equations, where u is assumed to be a function of two or more variables t (time), x and y (spatial coordinates).

A Linear Homogeneous Partial Differential Equation with

Homogeneous Linear PDE With Constant Coefficient- Method. Example Homogeneous equations Linear polynomial di erential operators In our example, y00+y0 6y = 0; with auxiliary polynomial P(r) = r2 +r 6; the roots of P(r) are r = 2 and r = 3. An equivalent. Higher Order Linear Di erential Equations Math 240 Linear DE Linear di erential operators Familiar stu Example Homogeneous equations :: Higher Order Linear Di erential Equations Math 240 Linear DE, Example Homogeneous equations Linear polynomial di erential operators In our example, y00+y0 6y = 0; with auxiliary polynomial P(r) = r2 +r 6; the roots of P(r) are r = 2 and r = 3. An equivalent. Higher Order Linear Di erential Equations Math 240 Linear DE Linear di erential operators Familiar stu Example Homogeneous equations :: Higher Order Linear Di erential Equations Math 240 Linear DE.

Classification of differential equations Maths for

Classification of differential equations Maths for. Examples 1. Partial differential equations A partial differential equation (PDE) is an equation giving a relation between a function of two or more variables, u,and its partial derivatives. The order of the PDE is the order of the highest partial derivative of u that appears in the PDE. APDEislinear if it is linear in u and in its partial derivatives. A linear PDE is homogeneous if all of, An example of a PDE: tension in the string In this case: = = = ρ ρ T T c2. Background to this course Partial Differential Equations Partial differentiation Ordinary Differential Equations Fourier series Numerical methods Vector calculus Electrical engineering Mechanical engineering Civil engineering Biomedical We now give brief reminders of partial differentiation, engineering ODEs, and.

The homogeneous Laplace equation, uxx + uyy = 0, can be thought of as a special case of the wave and heat equation where the function u(x,y,t) is independent of t. Nonhomogeneous differential equations are the same as homogeneous differential equations, except they can have terms involving only x (and constants) on the right side, as in this equation: You also can write nonhomogeneous differential equations in this format: y ” + p ( x ) y ‘ + q ( x ) y = g ( x ).

1 To determine the general solution to homogeneous second order differential equation: y " p (x )y ' q (x)y 0 Find two linearly independent solutions The homogeneous Laplace equation, uxx + uyy = 0, can be thought of as a special case of the wave and heat equation where the function u(x,y,t) is independent of t.

Partial Differential Equations Following are some classical partial differential equations, where u is assumed to be a function of two or more variables t (time), x and y (spatial coordinates). 1 To determine the general solution to homogeneous second order differential equation: y " p (x )y ' q (x)y 0 Find two linearly independent solutions

We study a homogeneous partial differential equation and get its entire solutions represented in convergent series of Laguerre polynomials. Moreover, the formulae of the order and type of the solutions are established. In this paper, we concentrate on the following partial differential equation (PDE Second Order Differential Equations is constant coefficient and homogeneous. In this example the dependent variable is x. (d) is constant coefficient and homogeneous. Note: Acomplementaryfunction is the generalsolution of ahomogeneous, lineardifferential equation. HELM (2008): Section 19.3: Second Order Differential Equations 31. 2. Finding the complementary function …

In order to solve this equation, let's consider that the solution to the homogeneous equation will allow us to obtain a system of basis functions that satisfy the given boundary conditions. We start with the Laplace equation: u x x + u y y = 0 . {\displaystyle u_{xx}+u_{yy}=0~.} Partial Differential Equations – the unknown function depends on more than one independent variable; as a result partial derivatives appear in the equation. Order of Differential Equations – The order of a differential equation (partial or

Partial Differential Equations This chapter introduces basic concepts and definitions for partial differential equations (PDEs) and solutions to a variety of PDEs. A linear partial di erential equation is non-homogeneous if it contains a term that does not depend on the dependent variable. For example, consider the wave equation

We study a homogeneous partial differential equation and get its entire solutions represented in convergent series of Laguerre polynomials. Moreover, the formulae of the order and type of the solutions are established. In this paper, we concentrate on the following partial differential equation (PDE Some Application of Partial Differential Equations In the are of Biology, Chemistry, Physics, Computer science (particularly in relation to image processing and graphics) and in economics (finance).

Examples 1. Partial differential equations A partial differential equation (PDE) is an equation giving a relation between a function of two or more variables, u,and its partial derivatives. The order of the PDE is the order of the highest partial derivative of u that appears in the PDE. APDEislinear if it is linear in u and in its partial derivatives. A linear PDE is homogeneous if all of example the pendulum equation, (1.1) d2Θ dt2 + g L sinΘ = 0, describes the angle, Θ, a pendulum makes with the vertical as a function of time, t. Here g and L are constants (the acceleration due to gravity and length of the pendulum respectively), tis the independent variable and Θ is the dependent variable. This is an ODE because there is only one independent variable, here t which

Second Order Differential Equations is constant coefficient and homogeneous. In this example the dependent variable is x. (d) is constant coefficient and homogeneous. Note: Acomplementaryfunction is the generalsolution of ahomogeneous, lineardifferential equation. HELM (2008): Section 19.3: Second Order Differential Equations 31. 2. Finding the complementary function … Nonhomogeneous differential equations are the same as homogeneous differential equations, except they can have terms involving only x (and constants) on the right side, as in this equation: You also can write nonhomogeneous differential equations in this format: y ” + p ( x ) y ‘ + q ( x ) y = g ( x ).

Second Order Differential Equations is constant coefficient and homogeneous. In this example the dependent variable is x. (d) is constant coefficient and homogeneous. Note: Acomplementaryfunction is the generalsolution of ahomogeneous, lineardifferential equation. HELM (2008): Section 19.3: Second Order Differential Equations 31. 2. Finding the complementary function … 20.pdf - Free download as PDF File (.pdf), Text File (.txt) or read online for free. Scribd is the world's largest social reading and publishing site. Search Search

LINEAR HOMOGENEOUS PARTIAL DIFFERENTIAL EQUATIONS

Homogeneous Linear PDE With Constant Coefficient- Method. In order to solve this equation, let's consider that the solution to the homogeneous equation will allow us to obtain a system of basis functions that satisfy the given boundary conditions. We start with the Laplace equation: u x x + u y y = 0 . {\displaystyle u_{xx}+u_{yy}=0~.}, Partial Differential Equations – the unknown function depends on more than one independent variable; as a result partial derivatives appear in the equation. Order of Differential Equations – The order of a differential equation (partial or.

PARTIAL DIFFERENTIAL EQUATIONS mat.iitm.ac.in. Second Order Differential Equations is constant coefficient and homogeneous. In this example the dependent variable is x. (d) is constant coefficient and homogeneous. Note: Acomplementaryfunction is the generalsolution of ahomogeneous, lineardifferential equation. HELM (2008): Section 19.3: Second Order Differential Equations 31. 2. Finding the complementary function …, The homogeneous Laplace equation, uxx + uyy = 0, can be thought of as a special case of the wave and heat equation where the function u(x,y,t) is independent of t..

LINEAR HOMOGENEOUS PARTIAL DIFFERENTIAL EQUATIONS

Partial Differential Equations engr.usask.ca. is an example of a partial differential equation. In this module we will only be dealing with Ordinary Differential Equations – The Basics Given an ODE in terms of dx dy , where y is called the dependent variable and x is the independent variable, our aim is to determine y in terms of x, without any derivatives. Consider the simple ODE = x2 − 1 dx dy. [5] The solution we desire will be Nonhomogeneous differential equations are the same as homogeneous differential equations, except they can have terms involving only x (and constants) on the right side, as in this equation: You also can write nonhomogeneous differential equations in this format: y ” + p ( x ) y ‘ + q ( x ) y = g ( x )..

Partial Differential Equations engr.usask.ca

Change of Variables / Homogeneous Differential Equation

PARTIAL DIFFERENTIAL EQUATIONS mat.iitm.ac.in

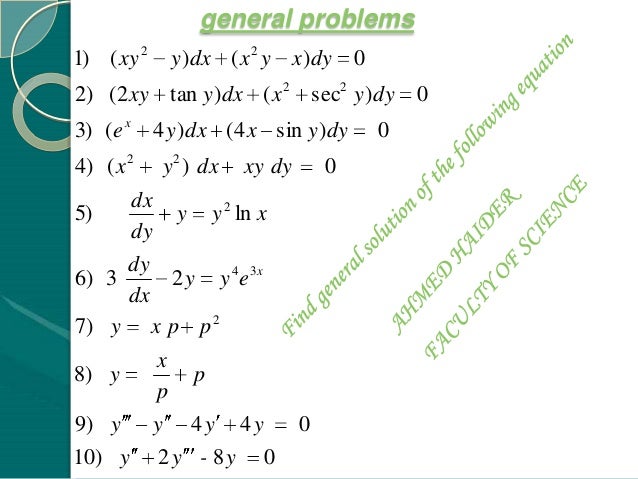
example the pendulum equation, (1.1) d2Θ dt2 + g L sinΘ = 0, describes the angle, Θ, a pendulum makes with the vertical as a function of time, t. Here g and L are constants (the acceleration due to gravity and length of the pendulum respectively), tis the independent variable and Θ is the dependent variable. This is an ODE because there is only one independent variable, here t which GEOMETRY OF A SYSTEM OF LINEAR HOMOGENEOUS PARTIAL DIFFERENTIAL EQUATIONS By Raymundo A. Fauila Introduction Using a system of partial differential equations, in this paper we shall study a configuration of surfaces and congruences of lines

The best solution strategy for differential equations depends on their order and whether they are ordinary or partial, linear or non-linear, and homogeneous or heterogeneous. The homogeneous Laplace equation, uxx + uyy = 0, can be thought of as a special case of the wave and heat equation where the function u(x,y,t) is independent of t.

Partial Differential Equations This chapter introduces basic concepts and definitions for partial differential equations (PDEs) and solutions to a variety of PDEs. An example of a PDE: tension in the string In this case: = = = ПЃ ПЃ T T c2. Background to this course Partial Differential Equations Partial differentiation Ordinary Differential Equations Fourier series Numerical methods Vector calculus Electrical engineering Mechanical engineering Civil engineering Biomedical We now give brief reminders of partial differentiation, engineering ODEs, and

Homogeneous PDE: If all the terms of a PDE contains the dependent variable or its partial derivatives then such a PDE is called non-homogeneous partial differential equation or homogeneous otherwise. In the above six examples eqn 6.1.6 is non-homogeneous where as the first five equations are homogeneous. 1 Introduction 1.1 Preliminaries A partial differential equation (PDE) describes a relation between an unknown function and its partial derivatives.

Homogeneous PDE: If all the terms of a PDE contains the dependent variable or its partial derivatives then such a PDE is called non-homogeneous partial differential equation or homogeneous otherwise. In the above six examples eqn 6.1.6 is non-homogeneous where as the first five equations are homogeneous. The homogeneous Laplace equation, uxx + uyy = 0, can be thought of as a special case of the wave and heat equation where the function u(x,y,t) is independent of t.

20/06/2011В В· In this video, I solve a homogeneous differential equation by using a change of variables. This example is looooong, requiring partial fractions to integrate! So much fun though! In order to solve this equation, let's consider that the solution to the homogeneous equation will allow us to obtain a system of basis functions that satisfy the given boundary conditions. We start with the Laplace equation: u x x + u y y = 0 . {\displaystyle u_{xx}+u_{yy}=0~.}

Partial Differential Equations This chapter introduces basic concepts and definitions for partial differential equations (PDEs) and solutions to a variety of PDEs. An example of a PDE: tension in the string In this case: = = = ПЃ ПЃ T T c2. Background to this course Partial Differential Equations Partial differentiation Ordinary Differential Equations Fourier series Numerical methods Vector calculus Electrical engineering Mechanical engineering Civil engineering Biomedical We now give brief reminders of partial differentiation, engineering ODEs, and

Examples 1. Partial differential equations A partial differential equation (PDE) is an equation giving a relation between a function of two or more variables, u,and its partial derivatives. The order of the PDE is the order of the highest partial derivative of u that appears in the PDE. APDEislinear if it is linear in u and in its partial derivatives. A linear PDE is homogeneous if all of An example of a PDE: tension in the string In this case: = = = ρ ρ T T c2. Background to this course Partial Differential Equations Partial differentiation Ordinary Differential Equations Fourier series Numerical methods Vector calculus Electrical engineering Mechanical engineering Civil engineering Biomedical We now give brief reminders of partial differentiation, engineering ODEs, and

7/11/2015 · This video lecture " Homogeneous Linear Partial Differential Equation With Constant Coefficient- CF and PI in Hindi" will help students to understand following topic of unit-IV … Partial Differential Equations This chapter introduces basic concepts and definitions for partial differential equations (PDEs) and solutions to a variety of PDEs.

Partial Differential Equations This chapter introduces basic concepts and definitions for partial differential equations (PDEs) and solutions to a variety of PDEs. Second Order Differential Equations is constant coefficient and homogeneous. In this example the dependent variable is x. (d) is constant coefficient and homogeneous. Note: Acomplementaryfunction is the generalsolution of ahomogeneous, lineardifferential equation. HELM (2008): Section 19.3: Second Order Differential Equations 31. 2. Finding the complementary function …

The best solution strategy for differential equations depends on their order and whether they are ordinary or partial, linear or non-linear, and homogeneous or heterogeneous. A linear partial di erential equation is non-homogeneous if it contains a term that does not depend on the dependent variable. For example, consider the wave equation